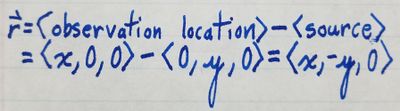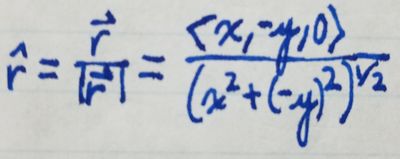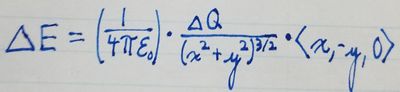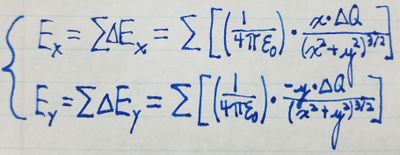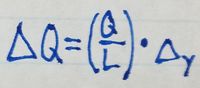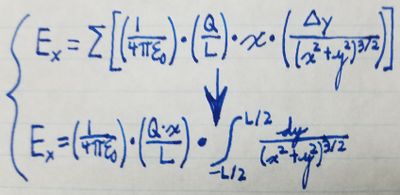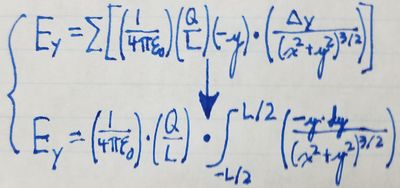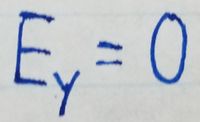Main Page: Difference between revisions
LukasYoder (talk | contribs) |
LukasYoder (talk | contribs) |
||
| Line 504: | Line 504: | ||
===Week 4=== | ===Week 4=== | ||
<div class="toccolours mw-collapsible mw-collapsed"> | <div class="toccolours mw-collapsible mw-collapsed"> | ||
=Field of a Charged Rod= | |||
''' | '''This entire page and all its contents were created by Lukas Yoder, PHYS 2212 Class of Fall 2018''' | ||
=== The Main Idea === | |||
Previously, we've learned about the electric field of a point particle. Often, when analyzing physical systems, it is the case that we're unable to analyze each individual particle that composes an object and need to therefore generalize collections of particles into shapes (in this case, a rod) whereby the mathematics corresponding to electric field calculations can be simplified. This can essentially be done by adding up the contributions to the electric field made by parts of an object, approximating each part of an object as a point charge. | |||
=== The System in Question === | |||
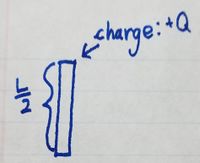
As discussed in the previous section, we're considering a system | |||
abstracted from the particle model we're familiar with, therefore we will | |||
make the generalization that our rod of length L has a total charge of | |||
quantity Q. For this generalization, we will need to assume that the rod | |||
is so thin that we can ignore its thickness. | |||
[[Image:LukasYoder01.jpg|200px|center]] | |||
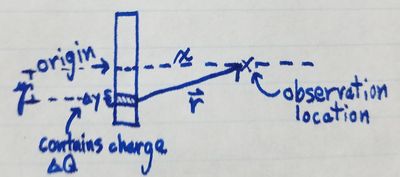
Since the electric field produced by a charge at any given location is | |||
proportional to the distance from the charge to that location, we will | |||
need to relate the observation location to the source of the charge, which | |||
we will consider the origin of the rod. To do that, we will need to divide | |||
the rod into pieces of length \delta y each containing a charge \delta Q. | |||
In the image below, you can see what this looks like and the relation that | |||
can be found between the observation location and the source, forming the | |||
distance vector \vect{r}. | |||
[[Image:LukasYoder02.jpg|400px|center]] | |||
By the pythagorean theorem, we can find the vector \vect{r} as follows: | |||
[[Image:LukasYoder03.jpg|400px|center]] | |||
And to find the unit vector in the direction of \vect{r}, \hat{r}, we do as | |||
follows: | |||
[[Image:LukasYoder04.jpg|400px|center]] | |||
=== Finding the Contribution of Each Piece to the Electric Field === | |||
Now that we've set up a model for the system, with the rod broken down | |||
into pieces, we can find the contribution of each piece to the electric | |||
field of the system. We will start from the electric field equation you | |||
learned for a point particle but plug in the parameters for the rod system | |||
into the equation. | |||
[[Image:LukasYoder05.jpg|400px|center]] | |||
By mathematically simplifying, we then get the following equation: | |||
[[Image:LukasYoder06.jpg|400px|center]] | |||
=== Finding the Net Contribution of all Pieces === | |||
In the previous section, we found out the contribution to the electric | |||
field at a given location of only one of the pieces constituting the rod. | |||
In order to figure out the net field at any particular location, we need | |||
to add up the electric fields produced by individual pieces along the | |||
length of the rod. | |||
We will switch from vector notation for the electric field to the scalar | |||
notation for the x- and y-components. (From the vector in the equation | |||
above, we can see that the z-component of the electric field at any point | |||
is always 0.) The x-component of the electric | |||
field is the sum of the x-components of every \delta{y} along the rod, and | |||
the y-component of the electric field is the sum of the y-components of | |||
every \delta{y} along the rod. We can show this mathematically: | |||
[[Image:LukasYoder07.jpg|400px|center]] | |||
To make use of this relation, because we don't know \delta{Q}, we need to | |||
relate it to parameters that we already know about the rod system we're | |||
analyzing. We can express \delta{Q} as the charge density of the rod | |||
(which is Q/L) times the \delta{y} we've chosen for the system. Thus, | |||
[[Image:LukasYoder08.jpg|200px|center]] | |||
By plugging the above equation into our equations for the x- and | |||
y-components of the electric field at a point, we can find the electric | |||
field at any point in the system. This technique is called numerical | |||
integration and is typically done by computers because the computational | |||
complexity is dependant upon the size of \delta{y} with respect to L. | |||
=== Simplifying === | |||
Using calculus, we can simplify a lot of the math required to compute the | |||
electric field at any given point. Notationally, all we're doing is switching from the | |||
discretely-sized \delta{y} to \textit{dy} and from the sigma notation to | |||
an integral starting from -L/2 (the lower end of the rod) and ending at | |||
L/2 (the upper end of the rod) as follows: | |||
[[Image:LukasYoder09.jpg|400px|center]] | |||
[[Image:LukasYoder10.jpg|400px|center]] | |||
By evaluating the integral, we can determine that the x-component of the | |||
electric field at any point is: | |||
[[Image:LukasYoder11.jpg|400px|center]] | |||
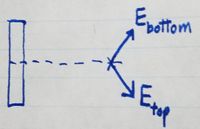
Without evaluating the integral for the y-component of the electric field, | |||
we can use symmetry to determine that the y-component of the electric | |||
field at any given point is 0. Let's consider the contributions to the | |||
electric field from the top and bottom halves of the rod at any | |||
observation point. | |||
[[Image:LukasYoder12.jpg|200px|center]] | |||
Since the y-components of E_top and E_bottom are of equal magnitude and | |||
opposite direction, they cancel each other out, and therefore the | |||
y-component of teh electric field at any given point due to the rod is 0. | |||
[[Image:LukasYoder13.jpg|200px|center]] | |||
Finally, because the rod is round and can be rotated, as a convenience, | |||
we'll use d (distance from the rod) as opposed to x (distance along the | |||
x-direction) to refer to the electric field. | |||
Thus we can simplify electric field calculations for a rod into a form | |||
that we can readily use: | |||
[[Image:LukasYoder14.jpg|400px|center]] | |||
=== Further Simplification === | |||
By noting the contributions of each variable to the equation for the | |||
electric field, we can make approximations to simplify our math by simply | |||
declaring one variable as insignificant. | |||
For example, if we have a system in which the length of a rod is much | |||
greater than the magnitude of the distance from the rod (denoted L>>d), we | |||
can neglect some of the instances in which d is taken into account as | |||
follows: | |||
[[Image:LukasYoder15.jpg|400px|center]] | |||
=== Finding the Electric Field from a Rod with Code === | |||
Here is some code that you can run which shows the electric field vector | |||
at a given distance from the rod along its length. The rod is shown as a | |||
series of green balls to help emphasize that when using the numerical | |||
integrations mentioned on this page, you are measuring the field produced | |||
by discrete parts of the rod being analyzed. | |||
Notice the edge-effects of the electric field of the rod. For reasons | |||
discussed above, if we used the long rod approximation (L>>d), these | |||
effects would be negligible. | |||
[http://www.glowscript.org/#/user/yoderlukas/folder/Public/program/ElectricFieldAlongRodLength Click Here to Run the Code] | |||
=== The Main Idea === | === The Main Idea === | ||
Revision as of 23:32, 25 November 2018
Georgia Tech Student Wiki for Introductory Physics.
This resource was created so that students can contribute and curate content to help those with limited or no access to a textbook. When reading this website, please correct any errors you may come across. If you read something that isn't clear, please consider revising it for future students!
Looking to make a contribution?
- Pick one of the topics from intro physics listed below
- Add content to that topic or improve the quality of what is already there.
- Need to make a new topic? Edit this page and add it to the list under the appropriate category. Then copy and paste the default Template into your new page and start editing.
Please remember that this is not a textbook and you are not limited to expressing your ideas with only text and equations. Whenever possible embed: pictures, videos, diagrams, simulations, computational models (e.g. Glowscript), and whatever content you think makes learning physics easier for other students.
Source Material
All of the content added to this resource must be in the public domain or similar free resource. If you are unsure about a source, contact the original author for permission. That said, there is a surprisingly large amount of introductory physics content scattered across the web. Here is an incomplete list of intro physics resources (please update as needed).
- A physics resource written by experts for an expert audience Physics Portal
- A wiki written for students by a physics expert MSU Physics Wiki
- A wiki book on modern physics Modern Physics Wiki
- The MIT open courseware for intro physics MITOCW Wiki
- An online concept map of intro physics HyperPhysics
- Interactive physics simulations PhET
- OpenStax intro physics textbooks: Vol1, Vol2, Vol3
- The Open Source Physics project is a collection of online physics resources OSP
- A resource guide compiled by the AAPT for educators ComPADRE
- The Feynman lectures on physics are free to read Feynman
- Final Study Guide for Modern Physics II created by a lab TA Modern Physics II Final Study Guide
Resources
- Commonly used wiki commands Wiki Cheatsheet
- A guide to representing equations in math mode Wiki Math Mode
- A page to keep track of all the physics Constants
- A page for review of Vectors and vector operations
- A listing of Notable Scientist with links to their individual pages